2017 センター試験 数学 I・A 第五問

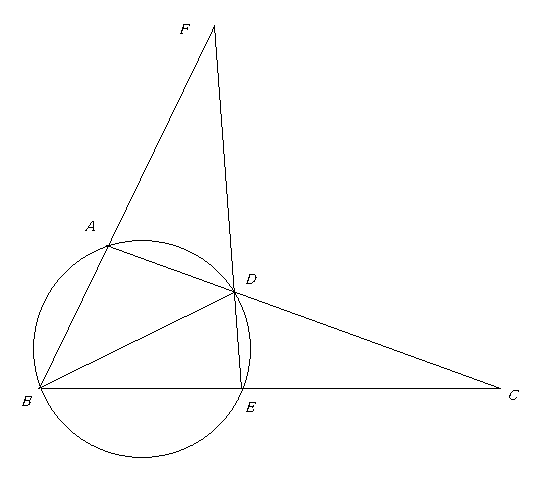
(1) 方冪 (べき) の定理より
$BC\cdot CE = AC\cdot CD = 7\times(7 - 3) = 28$.
$CE = \ds{28\over BC} = \ds{28\over8} = \ds{7\over2}$.

第五問 (1) の図

Menelaus の定理により
$${BF\over AF}\cdot{CE\over BE}\cdot{DA\over CD} = 1.$$

\begin{align} {BF\over AF} &= {BE\over CE}\cdot {CD\over DA} = {\ \ds{9\over2}\ \over\ds{7\over2}}\cdot{4\over3} \\ &= {9\over7}\cdot{4\over3} = {3\times4\over7} = {12\over7}. \end{align}

よって $AF = \ds{7\over5}BF = \ds{7\over5}\times3 = \ds{21\over5}$.

(2) (第二) 余弦定理より
$AC^2 = BC^2 + AB^2 - 2AB\cdot BC\cos\angle ABC$
$7^2 = 8^2 + 3^2 - 2\times8\times3\cos\angle ABC$

\begin{align} \cos\angle ABC &= {8^2 + 3^2 - 7^2\over 2\times8\times3} = {64 + 9 - 49\over 2\times8\times3} \\ &= {64 - 40\over2\times8\times3} = {24\over2\times8\times3} = {1\over2}. \end{align}

$0^\circ < \angle ABC < 180^\circ$ より $\angle ABC = 60^\circ$.

$r$ を内接円の半径とすると
$\ds{1\over2}\times3\times8\sin60^\circ = \ds{1\over2}(3 + 7 + 8)r$.
$3\times8\times\ds{\sqrt3\over2} = 18r$.
$r = \ds{12\sqrt3\over18}=\ds{2\sqrt3\over3}$

内心 I は, 頂角を二等分する線の交点なので, 下の図の三角形で $\angle B$ は $30^\circ$ である。

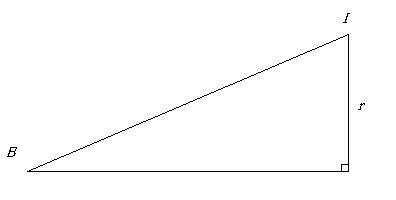
内心と頂角 B

従って $\ds{\ \ds{2\sqrt3\over3}\ \over BI} = \sin 30^\circ = \ds{1\over2}$ なので, $BI = \ds{4\sqrt3\over3}$.

(1) は Menelaus の定理だと気付くのに時間が掛かった。 ここは矢張り正確でなくとも良いから図を描かないと駄目。

(2) は, 最初, $BI$ を直接出す公式でもあるのかと思って焦ったが, 落ち着いて考えれば, それまでが hint になっていたのだった。